Mathematisches Institut
Auf der Morgenstelle 10
72076 Tübingen
Office: 2 P 31
Telephone: +49-7071-29-78596
E-mail: heller@mathematik.uni-tuebingen.de
 |
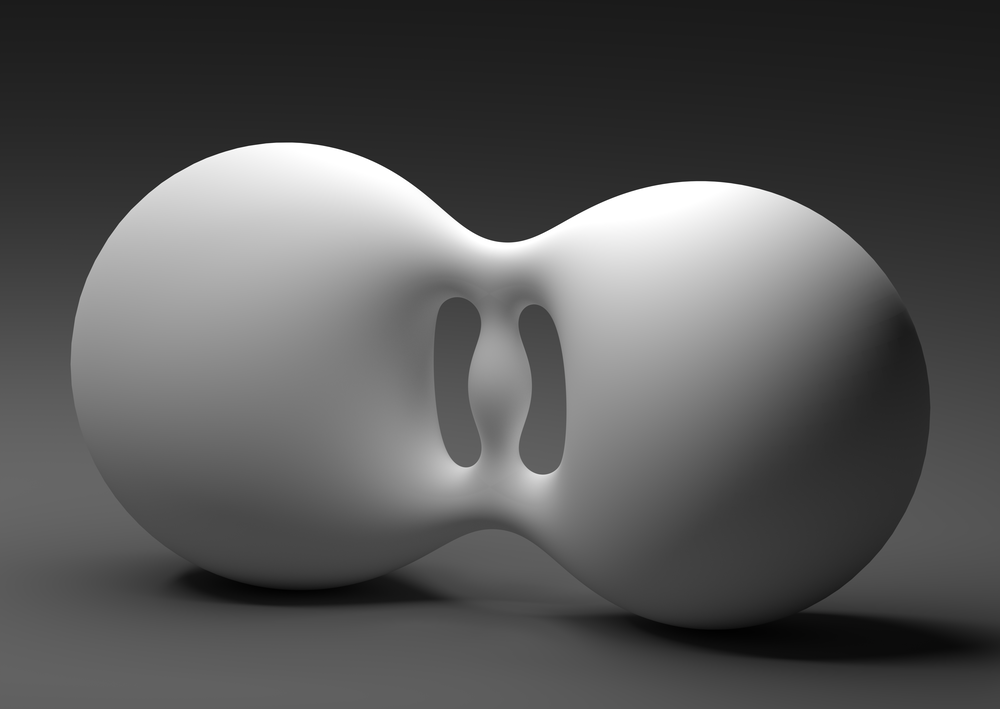 |
 |
Three views of a constant mean curvature surface in the 3-sphere. Images by Nick Schmitt based on the joint paper 'Deformations of symmetric CMC surfaces in the 3-sphere'.
Office Hours
open door policyTeaching
Winter term 2016/17:Riemannsche Flächen
Summer term 2016:
Ausgewählte Kapitel der Differentialgeometrie
Winter term 2015/16:
Mathematik für Physiker 3
Summer term 2015:
Mathematik für Physiker 2
Winter term 2014/15:
Ausgewälte Kapitel der Differentialgeometrie
Summer term 2014:
Seminar Geometrie von Flächen
Winter term 2013/14:
Einführung in Mannigfaltigkeiten
Seminar Riemannsche Flächen
Summer term 2013:
on parental leave
Winter term 2012/13:
Liegruppen und Liealgebren
Projektive Geometrie
Research
My research interests are global surface geometry, integrable systems and the geometry of moduli space of flat connections. I have developed a spectral curve theory for compact symmetric CMC surfaces of genus greater or equal two. Currently, I am generalizing the spectral curve theory to all CMC surfaces of genus 2. I am also studying a generalized Whitham flow on the spectral data of CMC surfaces which is used to construct new surfaces and to study the moduli space of CMC surfaces of higher genus.I am the principal investigator in the DFG programme "Constant mean curvature surfaces with non-abelian fundamental groups: theory and experiments".
Publications
-
The asymptotic behavior of the monodromy representations of the associated families of compact CMC surfaces
Bulletin of the London Mathematical Society 2016; doi: 10.1112/blms/bdw036
Preprint: arXiv:1505.00747 -
The spectral curve theory for (k,l)-symmetric CMC surfaces
with Lynn Heller and Nicholas Schmitt
J. Geom. Phys. 98 (2015), 201-213. http://dx.doi.org/10.1016/j.geomphys.2015.08.010
Preprint version -
Abelianization of Fuchsian Systems on a 4-punctured sphere and applications
with Lynn Heller
Journal of Symplectic Geometry, Vol. 14, No. 4 (2016), pp. 1059-1088
Preprint version Conformally flat circle bundles over surfaces
Diff. Geom. Appl. 40 (2015), 103-110.
Preprint version-
Deformations of symmetric CMC surfaces in the 3-sphere
with Nick Schmitt
Experimental Mathematics , Volume 24, Issue 1 (2015), pp 65-75.
Preprint: arxiv: 1305.4107 -
A spectral curve approach to Lawson symmetric CMC surfaces of genus 2
Math. Annalen, Volume 360, Issue 3 (2014), pp 607-652.
DOI: 10.1007/s00208-014-1044-4
Preprint: arxiv: 1209.3200 -
Lawson's genus two minimal surface and meromorphic connections
Math. Z., Volume 274 (2013), pp 745-760.
DOI: 10.1007/s00209-012-1094-9
Preprint version -
Higher genus minimal surfaces in S^3 and stable bundles.
J. Reine Angew. Math. (Crelle's Journal), Volume 685 (2013), pp 105-122.
DOI: 10.1515/crelle-2012-0011
Preprint version
Review -
Higher genus CMC surfaces via integrable systems
Abresch U, Pedit F, Umehara M.: Progress in Surface Theory. Oberwolfach Rep. Volume 10 (2013), pp 1253-1312.
DOI: 10.4171/OWR/2013/21
Conformal fibrations of S3 by circles.
Harmonic maps and differential geometry, Contemp. Math., Amer. Math. Soc., Providence, RI, Volume 542 (2011), pp 195-202.
Preprint version
ReviewHarmonic morphisms on conformally flat 3-spheres
Bull. Lond. Math. Soc., Volume 43 (2011), no. 1, 137-150.
Preprint versionGlobal aspects of integrable surface geometry
with A. Gerding, F. Pedit and N. Schmitt
in Systèmes intégrables et théorie des champs quantiques, eds: P. Baird, F. Hélein, J. Kouneiher, F. Pedit, V. Roubtsov, collection Travaux en Cours en Physique-Mathématiques, no 75, Hermann (2009).
Preprints
-
Branes through finite group actions
with Laura P. Schaposnik
Preprint: arxiv: 1611.00391 -
Exploring the space of compact symmetric CMC surfaces
with Lynn Heller and Nicholas Schmitt
Preprint: arxiv: 1503.07838 Navigating the Space of Symmetric CMC Surfaces
with Lynn Heller and Nicholas Schmitt
Preprint: arxiv: 1501.01929
Theses
Integrable systems methods for higher genus CMC surfaces in the 3-sphere
Habilitationsschrift, Universität Tübingen, 2013Conformal Submersions of the 3-sphere
PhD thesis, Humboldt Universität Berlin, 2008Articles in Preparation
Abelianization of flat special linear connections on n-punctured spheres (with C. Meneses)Integrable systems methods for CMC surfaces of genus 2